The deeper you drill into process capability analysis, the more you realize just how many capability indices are available. The two most common capability indices are Cpk and Ppk, and the closely related Cp, Cpu and Cpl, and Pp, Ppu and Ppl, respectively. Cpm is less commonly used, and has a unique way of accounting for the centeredness of a process within its specification limits. (See my past blog posts for extended discussions about all of these.)
Periodically though, you’ll also see mention of Cmk, often called “machine capability” or “initial process capability”. Thankfully, once you know how to use and interpret Cpk and Ppk, Cmk is easy to add to your repertoire.
Regardless of the abbreviations, all capability indices are based on the following general formula:
Process Capability = Specification Range / Process Range
The specification range is generally fixed and provided on the prints or engineering specifications for the part being produced. For instance, if your process is saw-cutting length of seamless pipe, the length tolerances and targets are likely found on your customer’s drawing or on a table of standard tolerances published by an industry association. These tolerances and targets are independent of the process.
The process range is determined by studying the process. Back to our saw-cutting example, combinations of the different saw types, blade types, fixture designs, coolants, etc. will produce varying process ranges. Some systems very tightly control the length variation, while others do not. By measuring the lengths of a sample of pipes, and then performing some statistical wizardry on the data points, you can estimate the process range–the length in which the great majority of all pipes will measure.
All of process capability analysis is about comparing these two ranges: What the customer expects to what the process can repeatedly produce.
For example, to calculate Ppk, a technician will generally collect at least 30 samples of a part drawn from a larger population, and measure the feature of interest on each sample. Using the collected data, Ppk is calculated with the following formula:

The upper and lower specification limits, USL and LSL, are found on the applicable engineering documents. The mean is the arithmetic average of the measured values. And “sigma”—the Greek letter that represents the sample standard deviation—is calculated using the Excel formula:
![]()
Ppk is then equal to the ratio of 1) the distance from the process mean to the nearest specification limit to 2) half the process range, denoted at 3 * sigma.
Now here’s the surprise: the formulas for Cmk is identical:
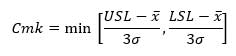
where σ = stdev.s(x1, x2, … xn)
The difference between Ppk and Cmk is not in the math, but in the process of drawing samples for measurement.
As mentioned earlier, Cmk is commonly referred to as “initial process capability”. Cmk is often used during the qualification of a new piece of equipment to determine whether or not it has the potential of meeting the part’s specifications. Where studies to compute Cpk and Ppk draw samples from a larger population to estimate how a process behaves over a longer period of time, a Cmk study is designed to minimize part-to-part variation thus isolating the inherent machine variation.
Once a process is set-up and running stably, a technician will draw consecutive samples—usually 50 or more—without making any adjustments to the machine or process. The feature(s) in question are then measured on each sample and the resulting data points are fed into the Cmk equations above. A plot of the individual data points on an I&MR control chart is also helpful to visualize the movements of the process outputs.
Like the more popular indices, Cmk also has a corollary, Cm, that does not account for centeredness. As you might expect, its formula is identical to Cp and Pp:

Interpreting Cm and Cmk is also identical in that: 1) Higher is better, and 2) a 1.0 means that the process exactly covers the full range allowed by the specification limits. Since a Cmk study is designed to minimize variation, a minimum of 1.67 or even a 2.00 is typically required to approve a machine or process. This extra confidence will help assure, that over time and the full range of process variables, the machine will still remain capable of repeatedly producing your part.
For an in-depth presentation of all thing Process Capability Analysis, sign up for my online class of the same name. I keep the selling price of the class low to allow as many quality professionals as possible to advance their skillsets and ultimately their careers. Click here to sign up.



